3D visualisation tools

Home Orange Blue Green Pink

3D visualisation tools

Installation notes
These demos have been programmed using LibQGLViewer, and QT 2.3 (Non commercial version).
They will work on windows XP.
What you need to do is :

1/ Copy these DLLs to your %WinDir%\System directory :
HERElogo

The OpenGL Utility Toolkit (GLUT) dll,

This file can also be found here
HERElogo

The LibQGLViewer dll,

This file can also be found here
HERElogo

The QT 2.3 dll,

This file came with the installation of the free non commercial QT 2.3 software,
HERElogo

An installer for this software. (not necessary to run the demos)

Also found here.

Instead of copying these files from their original locations, you can also save yourself time and just download :
ZIPlogo

this zip, which contains all the necessary DLLs to run the demos.

2/ Copy the .exe and the associated StateFiles presented below to a folder of your choice. The state file controls some default variables such as background color, window size... You can try to edit this file if you want.

3/ Execute the exe. Hit 'H' for help.

Demos

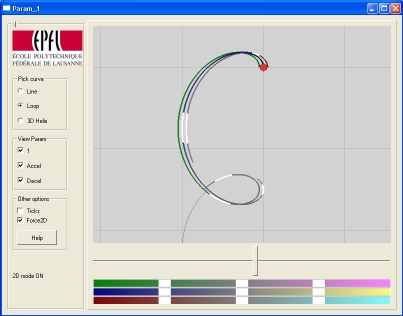	This demo illustrates curve parameterisation concepts. A slider controls the parameter value. The variation of this parameter draws a curve in 2D or 3D space. Several curves and different parameterisations of the same curves are available to visualise. Hit 'H' for more information on controls. Here.
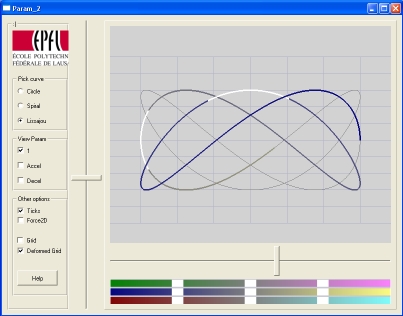	This demo illustrates curve parameterisation concepts too. A slider controls the parameter value. The variation of this parameter draws a curve in 2D space. Several curves and different parameterisations of the same curves are available to visualise. A second slider controls the scaling applied to one of the parameterisation components, showing how acting on the parameterisation affects the curve. Hit 'H' for more information on controls. 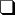Here.
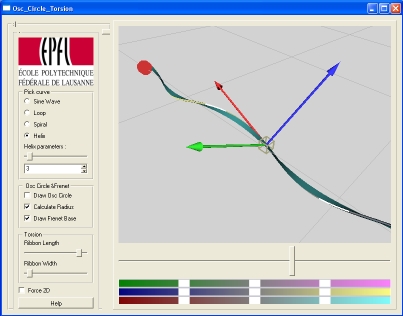	This demo illustrates curve tageant vectors, normal vectors, osculating circle, Frenet base and torsion. Pick a parameterised curve in 2D or 3D and a parameter value (bottom slider). Experiment with a tangeant circle (left slider) to find what radius seems to fit the best. Verify that the circle that best fits the curve is the osculating circle. Visualise the Frenet base (Tangeant vector, normal vector pointing to the osculating circle center, and third vector). Visualise the torsion (twisting "ribbon" fit to curve). Hit 'H' for more information on controls. Here.
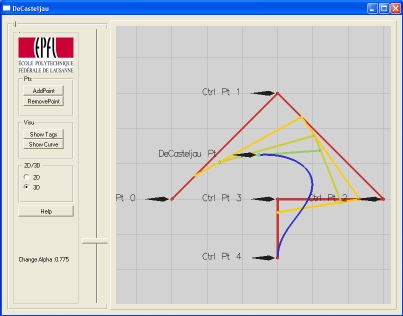	This demo illustrates the building and parameterisation of a Bezier curve. Add control points, select control points, manipulate them in 2D or 3D. Pick a parameter value and visualise the Bezier curve point corresponding to this value found by the DeCasteljau algorithm. Hit 'H' for more information on controls. Here.

Home Orange Blue Green Pink